Mesopotamian Science and Technology - I
last update: 20 Nov. 2019
These three webpages have been built from more than 100 books, scientific papers, chapters, and websites (some are referenced in the text, and others at the end of the third webpage). I've proof read the text and there is inevitably some repetition, possibly just enough, possibly too much. I have written these pages for my own personal interest, but I welcome comments from anyone who might, by chance, happen upon these pages.
So these three webpages are a bit of an experiment. What I would like to do here is include a very short history of science and technology (natural sciences) as seen before ancient, classical and Hellenistic Greece. First, I’m not sure if its possible to do this in a useful and intelligent way. Secondly, I’m not sure I can do it justice.
Looking at Mesopotamia means looking back into the very remote past of what we might call 'intelligent' modern Man. In fact Mesopotamia is one of the great civilisations of antiquity, and consists of a sequence of intertwined cultures revealed though a considerable body of material provided by numerous excavations at a wide variety of sites.
My aim is always to look at the emergence of the natural sciences, but often I will admit to not being able to extract a theory or a deep understanding from the description of a technology or technique. However I've tried to describe those technologies and techniques as best as I can, and I hope my narrative inspires others to delve more deeply into this fascinating history.
Here are a few general purpose sources you can check out:
The Chronology of the Ancient Near East (ca. 3700-539 BC) and the Short Chronology Timeline (ca. 1728-1531 BC)
The List of Mesopotamian Dynasties (ca. 2500-539 BC), along with Sumerian King List (ca. 2700-1730 BC), List of Assyrian Kings (ca. 1905-608 BC), List of Kings of Akkad (ca. 2334-2022 BC), List of Hittite Kings (ca. 2200-1178 BC), List of Neo-Hittite Kings (ca. 1321-640 BC), List of Rulers of Mitanni (ca. 1500- 1263 BC), and the List of Kings of Babylon (ca. 1961-141 BC)
List of Rulers of the Pre-Achaemenid Kingdoms of Iran (ca. 2700-530 BC) and the List of Rulers of Elam (ca. 2700 BC to 224 AD)
Cities of the Ancient Near East
History of Mesopotamia and the History of Sumer
I have prepared three webpages.
This first webpage covers the history of writing and numbers (including cuneiforms) and astronomy (including lists of omens).
The second webpage covers the so-called “science of crafts” with cave painting, tool making (including stone tools), fire and pre-pottery technologies, weaving and textiles (ca. 60,000 BC), ceramic sculptures and pottery (starting ca. 29,000 BC), boats (ca. 10,000 BC), the wheel (ca. 6500 BC), and concludes with early patterns of trade and maps (ca, 12,000 BC).
The third webpage covers irrigation, and metallurgy of the Copper, Bronze and Iron Ages.
It is worthwhile keeping in mind some of the 'periods' that make up Mesopotamian history:
Natufian Culture (ca. 13,000-7500 BC)
Neolithic "New Stone Age" (ca. 10,000-4500 BC)
Pre-Pottery Neolithic (somewhere between 10,000-5500 BC)
Pre-Pottery Neolithic A (ca. 9500-8000 BC)
Pre-Pottery Neolithic B (ca. 7600-6000 BC)
Pottery Neolithic (ca. 7000-5500 BC)
Hassunah Period with Hassuna Culture ca. 6900-6500 BC and Samarra Culture ca. 7000-4800 BC
Halaf Period (ca. 6500-5100 BC)
Ubaid Period (ca. 6200-4000 BC)
Chalcolithic "Copper Age" (ca. 5500-3000 BC)
Warka and Proto-Literate Periods (Uruk Period ca. 4100-3000 BC)
Gaura and Ninevite Periods (Tepe Gawra ca. 5000-1500 BC, Nineveh ca. 6000-612 BC)
Jemdet Nasr Period (ca. 3100-2900 BC)
Bronze Age (ca. 3300-1200 BC)
Early Bronze Age with Early Dynastic Period (ca. 2900-2350 BC), Akkadian Empire (ca. 2350-2150 BC), Third Dynasty of Ur (2112-2004 BC) and the Early Assyrian Kingdom (ca. 2600-2025 BC)
Middle Bronze Age with Early Babylonia (ca. 1900-1800 BC), First Babylonian Dynasty (ca. 1830-1531 BC), Empire of Hammurabi (ca. 1810-1750 BC) and Minoan eruption (c. 1620 BC)
Late Bronze Age with Old Assyrian period (2025-1378 BC), Middle Assyrian Period (c. 1392–934 BC), Kassites in Babylon, (c. 1595–1155 BC) and Late Bronze Age collapse (ca. 1200-1150 BC)
Iron Age with Syro-Hittite States (ca. 1180-700 BC), Neo-Assyrian Empire (911-609 BC) and Neo-Babylonian Empire (626-539 BC)
As you can guess from the above list, establishing a definitive stratigraphy on the way artefacts are 'layered' and dated on a particular site and how the context between layers is interpreted has always been an open issue in archaeology. In addition some terms such a Sumerian, Sumero-Akkadian, Assyro-Babylonian, etc. are often not precise in terms of geographic coverage or chronology (even spellings are different). Lists of time periods are notorious for all being different in different texts, and l have simply used a range of dates given on a variety of different sources. Individual authors are often very coherent in their definitions and chronology, but as I have done, when you move across multiple publications and websites you immediately realise that each uses a slightly different chronology to contextualise their research. The key here is that we are trying to discover features of Mesopotamian science and mathematics, not create a coherent description of the history of one of the world's earliest civilisations.
Below we have a simple map of the region indicating both present-day locations and some of the ancient sites that are mentioned in these three webpages.
Before we delve into the details, let's just remind ourselves about the topics that we will try to illustrate in these three webpages.
Mathematics has its origins in both Babylonian mathematics (ca. 1900 BC) and Egyptian mathematics (ca. 2000-1800 BC). All the writings of that period appear to be about the so-called Pythagorean theorem, and build-on the evolution of some basic arithmetics and geometry. Greek mathematics greatly refined the methods, introducing deductive reasoning and mathematical rigour and proofs. See also Chinese mathematics, the Hindu-Arabic numeral system, Islamic mathematics, and the Timeline of mathematics.
Science is said to have had its origins in Ancient Egypt with Egyptian astronomy (ca. 5000 BC), mathematics and Egyptian medicine (ca. 4000 BC). In Sumer (ca. 3500 BC) the Mesopotamian people studied the sciences, with Babylonian astronomy, mathematics, and Babylonian medicine. See also the History of Science in Early Cultures, Science and Technology in Ancient India (from 4000 BC in the Indus Valley), History of Science and Technology in China (from ca. 500 BC).
Law has its history in ancient Egyptian law, based upon the concept of Ma’at, and may date from 3000 BC. The first law code came from the Sumerian ruler, Ur-Nammu, and dates from ca. 2200 BC (although Urukagina of Lagash may have had a legal code as early as ca. 2400 BC). Around 1760 BC, King Hammurabi, further developed Babylonian law, which became known as Codex Hammurabi. Ancient Greek law can be dated back to the Draconian law code, ca. 621 BC. Roman law can be dated back to 449 BC with the so-called Twelve Tables. Traditional Chinese law probably dates from the Zhou Dynasty, ca. 1000 BC. The Yajnavalkya Smriti was a Hinduism legal text dating from as early at ca. 500 BC, and Manu Smriti was another Hinduism legal text that may have dated from ca. 200 BC (see Hindu Law and Classical Hindu Law).
Literature can be considered to have started with writing, and it has its root in both Bronze Age Mesopotamia and Ancient Egypt. Writing has its origins in Mesopotamia, especially ancient Sumer, around 3200 BC. The oldest known literary texts date from ca. 2700 BC, starting with the Sumerian texts from Abu Salabikh (dated ca. 2600 BC), Egyptian Pyramid Texts (dating from ca. 2400 BC), Akkadian Laws of Eshnunna (dated ca. 1950 BC), and including the Vedas, the sacred texts in Vedic Sanskrit (from sometime between ca. 1700-1100 BC). The oldest existing collection of Chinese texts is the Shijing, which dates from sometime between the 11th C and 7th C BC. See also Sumerian literature, Akkadian literature, Ancient Egyptian literature, Hittite texts, Chinese classics and Classical Chinese poetry.
Greek literature more or less kicks off with the works of Homer, the Iliad and the Odyssey. These so-called pre-classical texts date from ca. 800 BC (see Ancient Greek literature). The other great authors of the period was Hesiod, with his Works and Days, and Theogony (both dating from ca. 700 BC).
Mesopotamia is the name given to the Tigris-Euphrates river system, which during the Bronze Age (ca. 3300-1200 BC) was home to Sumerians (ca. 5300-1700 BC), Akkadians (ca. 2334-2154 BC), Babylonians (ca. 2334-1654 BC), Assyrians (ca. 2500-613 BC), and later to the Neo-Assyrian (911 BC-605 BC) and Neo-Babylonian (626-539 BC) peoples. In 539 BC the whole area was absorbed into the Achaemenid Empire (ca. 650-330 BC) or First Persian Empire, before being in turn absorbed by Alexander the Great (who died in Babylon in 323 BC). After his death Babylon became a province of the Persian Empires until 650 AD.
Often Babylonia and Babylonians are used as a generic term meaning Mesopotamia and the whole region covering modern-day Iraq, Syria, and regions on the Turkish-Syrian and Iran-Iraq boarders. For example, the Sumerians and Akkadians had two linguistically unrelated languages, but they shared the same syllabic script we now call cuneiform. This script, and the dried clay tablets on which it was written, evolved considerably through the Bronze Age, but we tend always to see it associated with the Babylonians and Babylon. And we rely on cuneiform sources for our understanding of all ancient Middle Eastern literate cultures. There are between 500,000 and 2,000,000 tablets held in the worlds museums, but most have never been viewed and translated. The majority of tablets deal with numbers (within administrative documents), but only a small number are useful in terms of the history of mathematics and the sciences (and most of those are multiplication tables). Oddly, this mathematics is treated as part of the 'Western' tradition, without there being much evidence of its influence on Classical (Greek) mathematics until after Euclid (active in 3rd C BC). If cuneiform writing looks interesting to you, check out the Cuneiform Digital Library Initiative.
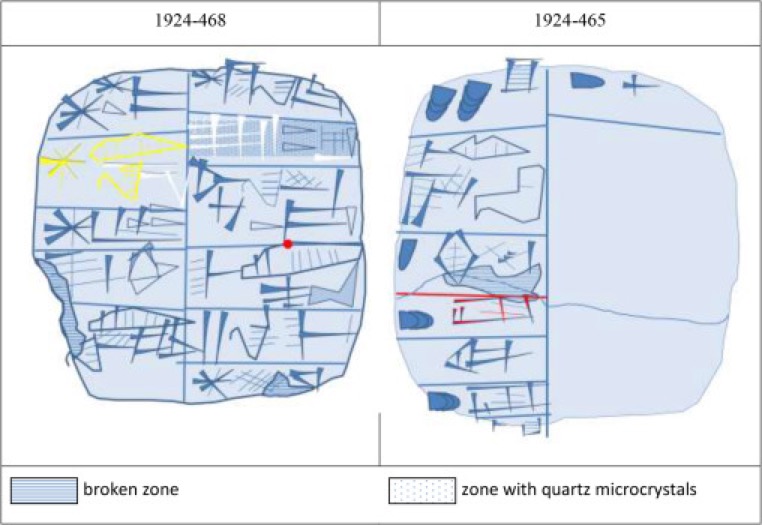
It has rightly been pointed out that the cuneiform script must owe much to pottery-firing technology which was widespread in the northern Iraq region from ca. 6000 BC. Potters used bands with finite designs, and developed uniform and precision skills. The key was perhaps “neat, busy, and abstract”. Cylinder seals, small round cylinders engraved with written characters, date from ca. 3500 BC, and were used to identify their owner, or define an institutional function. They were prepared as mirror images and used to create clay impressions to define some kind of ownership (the one below dates from ca. 2500-2400 BC). To see some more cylinder seals, check out the "Mesopotamian Cylinder Seals Collection" in the Spurlock Museum, Illinois.

It is true that very few of these cylinder seals display any direct mathematical inspiration, although some “stone carpets” (see below for an example from ca. 640-645 BC) do have what has been considered mathematical design inspiration, i.e. two-, four-, and even eight-fold symmetry, interlocking circles, shapes within shapes, stylised design.

Archaeogenetics of the Near East
Wikipedia has an article with this exact title, looking at the genetics of past human populations (archaeogenetics) in the Ancient Near East using DNA from ancient remains. Wikipedia tells us that researchers use Y-DNA, mtDNA and other autosomal DNA's to identify haplogroups and haplotypes in ancient populations of Egypt, Persia, Mesopotamia, Anatolia, Arabia, the Levant and other areas.
The reality is that the topic is complex and would merit its own webpage (which I hope to prepare one day), but we can summarise things as follows (taken from a series of webpages dedicated to the topic).
"Ancient DNA studies have shown that the Near East consisted of several distinct populations in the Early Neolithic period. The Natufian-derived population of the southern Levant had affinities with the Red Sea region (Y-haplogroup E1b1b). Anatolian tribes seem to have belonged primarily to Y-haplogroup G2a, who brought agriculture to Europe. South Caucasians would have belonged to Y-haplogroups J1 and J2, later associated with the Kura-Araxes culture. The Y-haplogroup T1a may have been found around Mesopotamia".
History of writing and numbers
During ca. 4000-3000 BC temples (and scribes) developed ways to manage offerings, and small clay counters (or tokens) with different shapes appeared to have represented fixed amounts of commodities.
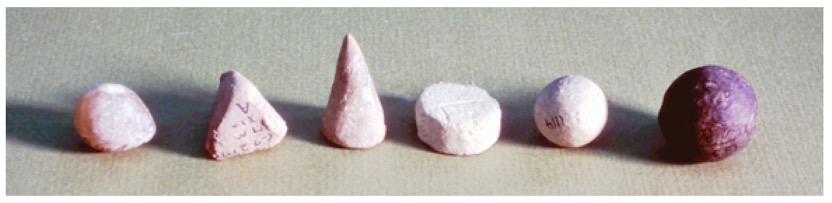
Some experts suggest that these tokens date back to ca. 7500 BC, but their purpose was only “solved” in 1969 (the ones above dates from ca. 4000 BC). Initially simple shapes, they evolved to more complex forms bearing markings. Each token represented a specific quantity of a specific commodity, the small cone was for a small measure of grain and a sphere for a large measure of grain. So each type of item was counted with its own numeration system and number words.
By ca. 3300 BC these small clay counters were kept in rough clay envelopes, with a counter mark on the external surface (the below envelop dates from ca. 3300 BC). The discs below it represented a 'flock' of animals. The big cone was for a very large measure of grain, and the small cones for small measures of grain.

It is presumed that at some moment in time the envelop was abandoned in favour of a simple tablet. The oldest clay (pre-arithmetical) tablets carry the impressions of these counters, with or without cylinder seals. Some tablets have the same counter impression repeated several times, others show a mix of different counter shapes and sizes. It is assumed that there was some implicit numerical significance to the tablets.

Here we have an impressed tablet from ca. 3200 BC. Each circular impression stood for one large measure of grain, and each wedge (cone) for a smaller measure of grain. The lighter impressions are official seals. The scribe-accountant would roll his cylinder seal all over the tablet or envelop, then he would make deeper mark with a token or stylus. By ca. 3100 BC some tablets simply stood for a number of items, with circular imprints for tens, and wedges for units. And in the corner you would have a depiction of the actual type of item.
Can we step back a moment to Man's earliest attempts at numbers. Experts often talk of numbers in terms of numerals as articulated in the so-called Proto-Indo-European language (possible post 10,000 BC), and they suggest that it was all based upon our fingers. But the earliest numerals (or perhaps counting aids is a better description) appear to be the tally marks in tally sticks (possibly dating to ca. 30,000 BC or even earlier).

These sticks just have repetitive marks on them, each notch corresponding to one unit. The numbers cannot be 'read' but must just be 'counted'. There is no arithmetic, and in fact we do not have any evidence that these marks were associated with a concept of a number. You can have six marks representing six days, without having the concept of the number six. One challenge for the early user of tally marks was how to represent part of one unit (fractions). How to represent large numbers, was another challenge. Experts have suggested that recognising small numbers (i.e. 1, 2, 3, ..) seems to be inborn, and that everything might have started by recognising that halving a certain number of items would leave either two or three items (even or odd). Here we have two useful introductions, firstly “Number Words and Arithmetic: A story of Numbers”, and secondly Number Systems and the Invention of Positional Notation.

So now back to our Babylonians. Sometime between 3300-3000 BC tablets appeared that mixed word signs (pictograms), counter shapes, and cylinder seals. The need to identify the object being numbered or accounted for was arguably a prime motivation behind the invention of the incised proto-cuneiform script (see proto-writing). The pictograms were scratched into the clay, and not impressed. They were known to be used in the management of the wealth of a temple, and some of the earliest tablets appear to be about different kinds of grain used to brew beer and make bread.
At the time there were at least a dozen different metrological systems (numerical systems) and bases in use, depending upon what was being accounted for, and if it was counting time, area, discrete objects, or quantities, or various grains or liquids. It has been suggested that because there were so many different metrological units, scribes simplified everything by converted all measures into sexagesimal multiplies and fractions of a basic unit, and used those numbers for calculations (there are indications that this was happening as early as ca. 2340 BC). Individual accountants and administrators were so important that their names appeared ca. 500 years before the first royal inscriptions. Between 3000-2100 BC incomes and expenditures (of the temple, of the city, and even of the richer families) started to be accounted for. It was during this period that the sexagesimal place-value system was invented, and by 2100 BC the law-code showed an increased tendency to sexagesimalisation across different metrologies.
The discovery of sexagesimal counting by the Babylonians actually dates from only 1854, and an understanding of their use of a place-value system and the functioning of their tables of reciprocals dates from 1915. An understanding of Babylonian metrology only emerged during the period 1872-1984, and brick metrology had to wait until 1915. Some experts claim that the difficulties in following the progress of the Babylonian number and accounting system was because scribes were instructed not to show their arithmetic workings. By ca. 1800 BC barley (representing small value items) and silver were the commercial exchange units, and the law-codes set out ideal rates of exchange, wages, and professional fees. Loan interest rates were 33% on barley and 20% on silver.
Much has been said on the evolution of mathematics as a means to measure fields and predict harvests. From ca. 3700 BC fields were elongated strips abutting to irrigation channels, and designed to be easy to plough using animals. Irregular shapes were divided into right triangles and irregular quadrilaterals. So area calculations were important in predicting crops and in the repartition of inheritances. From ca. 3500 BC allotted target work-rates were used as a unit of accounting (the working day was 10 hours, 365 days a year). See “The uses of mathematics in ancient Iraq, 6000-600 BC”, by Eleanor Robson, for more details.
Babylonian science
The trend is often to study the history of science by looking at the ancient Greeks and Romans, and by ignoring the Babylonians (and other even more distant cultures). We must start by understanding that the Babylonians had to be ingenious in exploiting their land and water resources, since they had little in the way of wood, stone, or metals. Due to their use of baked clay tablets we know something of their science and mathematics. In fact we know far more about the Babylonians then we know about ancient Egyptian science and mathematics, since papyrus only survived under exceptionally dry conditions. Almost all of the Babylonian tablets involved numbers, and were used to keep track of what people and institutions owned and owed. However, over time tablets were also used to record more abstract ideas about their world, for example, The Story of Gilgamesh was recorded on clay tablets (possibly as early as ca. 2100 BC). Some tablets were student-scribe exercises as they learned to write, reason, and calculate. Other tablets included day-to-day correspondence between scholars, and reports to patrons. In many ways cuneiform tablets provide a clearer insight into everyday life in Babylonia, as compared to Egypt, Greece, or even Rome. It is true that we only know about those places where archaeological digs have been made. And we know that the Babylonians also used papyrus, parchment, and wooden boards as writing surfaces, but all have disappeared. On the positive side, sometimes the tablets were found in specific contextual situations that helps in their decryption.
Babylonian numbers and mathematics

The most important difference with Babylonian numbers is that they were in base 60, and not base 10. They had nine unit signs, and five tens signs, so they were able to write up to 59. Then the numbers 1-59 could be put together to write any number needed. As with our system the change of position of a number next to another changed its meaning (positional notation). Babylonians would write 1 24 (= 84) and 4 21 (= 261), i.e. 1 sixty, plus 2 tens, plus 4 units, or 4 sixties, plus 2 tens, plus 1 unit. As with our numbering system, there was no upper or lower limit to Babylonian numbers. It is not clear why they decided on a numbering system to the base 60, but it has been suggested that 60 can be divided by 2, 3, 5, 10, 12, 15, 20, and 30. In any case the unit of 60 was adopted by the Greeks to measure angles and count out time. We still have today 360° in a circle, and 60 minutes in an hour.
There are examples of tablets dating from ca. 1750 BC, that are evidently mathematical problems to be solved (possibly by children or trainee scribes). There are some indications that once the problems were solved, the tablets were simply cut up into tiny bricks and used as building materials. It would appear that they started with learning to make the basic elements of cuneiform script, then on to signs, people’s names, names of objects, all in a standardised order. This so-called 'elementary' level involved a lot of repetition of huge lists. Then on to numbers, and weights and measures, e.g. multiplication tables (or tablets). This involved starting with metrological lists always in ascending order, and in order, capacity (e.g. grain), weight (e.g. silver), surface (e.g. field), and length, and was followed by metrological tables with written numbers. Length was an odd one, last in the list because it was not a generic term, you had 'length' for length, width, diagonal, height, and depth (and they had different metrological tables for horizontal and vertical dimensions). During this period metrological units were written out in ascending order, up to the equivalent of 65 million litres, 47,000 hectares, and 650 km. The numerical tables included reciprocals, multiplications, squares, square roots, and cube roots. For example, lists were made of wooden boats and measuring vats of different capacities. Different parts of weighing scales were learned, as was the use of stone weights and measuring-reeds. Weights and measures were learned along with the measuring equipment, never as an abstract system, and in this case always in descending order of size. Only then on to whole sentences and texts, and model letters, legal documents, and proverbs and sayings. Students would need to memorise long sequences of facts, and copy out about 40 standard tables for multiplications and reciprocals. Students would then learn algorithms for calculating multiplications, reciprocals, surfaces, and occasionally volumes. Tablets found in Nippur suggest that students were faced with at least, 187 metrological lists, 161 metrological tables, 417 numerical tables, and 38 calculation exercises. There are tablets with the teachers elegant writing on one side, and the pupils poor efforts on the other. On top of that you can see that some things were rewritten, and on the 'back' side of the tablet longer passages were practiced over and over again (giving a clear view of the standard order of learning). It is through these tablets that we can see how the Babylonians learned to employ mathematics and geometry, and thus we have an insight in to how civilisation mastered those topics. Below we have a typical Sumerian school tablet.

Then, after a long 'elementary' phase, the students would begin to learn mathematical facts by drawing geometric shapes and calculating their areas. Mathematics was clearly an essential part of the work of a scribe, since about half the tablets found in the schools were metrological texts. There were also texts describing the appropriate behaviour of professional scribes, and they also had to be copied and re-copied. There is a clear sense that good mathematical practices (and literacy) were expected to bring about peace and social justice. Mathematics was seen as a tool to ensure justice and fairness in the world. Scribes must work to uphold the legal system (e.g. inheritance, sales, harvest contracts, etc.), prevent disputes, or ensure that they were settled peacefully. We have images of Kings holding rulers and measuring ropes, a clear sign of the importance measurement and mathematics had. Writing, counting and calculating were tools for social justice. The Codex Hammurabi (dated 1754 BC) lists more than 280 laws concerning fair payment for goods and services, proportionate distribution of lands and inheritance, and punishments for transgressors.
Perhaps of more interest to us are the mathematical tablets. One of them gives us a good idea about the simple test problems used in training scribes. The problem was to extend an animal pen, given a set number of bricks to pave the new area. The question was how big was the new animal yard? The answer was a square of 20 cubits on each side (a Babylonian cubit was 51.86 cm). Everyone tends to gloss over the most basic of arithmetic tools, proportionality. One of the earliest questions, and a supra-utilitarian question at that, was simply “if 1 gú-bar is enough for 33 persons, how much do you count with for 260,000 persons?”. Normally one would use division to obtain an exact answer, but early Babylonians tried approximate proportions, i.e. 3 gú-bar for 99 persons, 30 gú-bar for 990 persons, and so on. This led to the development and use of the false position method, which was already in use by 1700 BC for 'complex' questions concerning finding the sides of rectangles if given the length of the diagonal. Experts have looked beyond this simple arithmetic procedure, to see a major shift in the thinking of Man. You can find a 'true' quantity through the use of a false quantity, and perhaps even more importantly you can think about your 'true' quantity through thinking about another quantity. Mesopotamian epic poems, literature and religious texts used metaphors constantly in order to get their 'true' message across. Some experts have detected in the use of the false position method the early algebraic thinking of the Babylonians. Scribes would often take '1' as their first false solution, much the same way as we take 'x' in modern algebraic procedures. The suggestion is that the '1' was another way of saying “I don’t know”, but there is no proof that this was true. They may simply have used '1' to systematise their numerical problem-solving methods. We do know that later scribes would just put in the sought-after object itself, e.g. 'width' instead of '1', and we thus really can see the emergence of algebraic thinking (an algebraic idea of an unknown rather than the earlier 'false' quantity).

We are now going to look very carefully at one specific clay tablet, YBC 7289, or tablet 7289 in the Babylonian Collection of Yale University. It maybe one small clay tablet, but it is probably the most famous Babylonian clay tablet in existence. The tablet dates from the Hammurabi dynasty, ca. 1800-1600 BC. It is a so-called hand-tablet (due to its small size) and appears to be a school exercise by a novice scribe. The numbers on the middle photograph are the actually translations of the cuneiform writing, recognising the use of Babylonian numbers (i.e. a sexagesimal system, or written in base 60), and the image on the right is just the back of the tablet. What we see in the numbers is in fact a numerical estimate of √2 expressed to 6 decimal places. It is quite possible that the scribe did not compute the number himself, but copied it from an existing table of values. We must remember that at that time Babylonians did not possess a 0, so the 30 could also mean one-half. I understand that the “nothing in this column”, a kind of 'zero' as a place holder, appeared sometime between 700-300 BC, but even then this 'zero' was never placed at the end of a number. Interpreting the full meaning of the tablet is open to discussion. But one credible interpretation is that (a) the Babylonians interpreted ratios of lengths as numbers, (b) that this was not just a good approximation, but they knew that the ratio of the diagonal of a square to a side was a number whose square was 2, and (c) that they possessed an algorithm for finding approximations to √2 (see this description). What we see is a dissection of the square on the hypotenuse of an isosceles right triangle into pieces which can be reassembled to make up the two squares on the sides. This has been claimed by some experts to be one of the first true examples of both visual reasoning, and, more impressively, reasoning itself. It is also the greatest known computational accuracy obtain anywhere in the ancient world (accurate to 5 decimal places).
One of the best sources of information on Babylonian mathematics is Mesopotamian Mathematics. Here we learn that we must look to a small number of tablets dating from around 3200-2900 BC to understand the emergence of numbers, and thus arithmetic. Some tablets show quantities of commodities and possible a title of a responsible official. Numbers were more probably just concepts of quantities, a bit like icons. More than 1200 archaic signs are known, but only about 60 were quantity signs. And these were arranged in about a dozen series, depending on what they were measuring. It would appear that a sexagesimal system was used to count objects (e.g. people, sheep), and a bisexagesimal system for counting discrete rations of objects, e.g. pieces of cheese. A separate different counting system was used for grain, and some symbols could have different meanings depending upon what they were counting. There is no real information on how computations were performed during this very early period.
During the period 2900-2350 BC the number of quantity signs was reduced, and their shapes became more regularised and abstract. New syllabic signs appeared, indicating the writing of the grammar of a spoken language. This meant that the cuneiform could be used to document history or even write literature. Number writing did evolve, but there are only a few indications on how arithmetic or mathematics evolved. We see the first multiplication table, and the first geometrical exercise (we do not know what the problem was). From this we know that mathematics was being taught from about 2500 BC. We also know that during this time the basic numerical sign system was reduced to a sexagesimal system and an area measuring system.
In the Sargonic period (ca. 2350-2200 BC) of the Akkadian Empire, numbers started to be written in cuneiform. We now have a dozen or so metro-mathematical tablets, and the problem of partitioning a trapezoid appears in the education of scribes. We know that reforms were put in place to facilitate calculation using the 'new' sexagesimal system, and concepts of area, volume, capacity, and weight were well defined. The Wikipedia article Ancient Mesopotamian units of measurement is particular interesting in this context.
In the period 2100-2000 BC they introduced a new system of weights and measures, and a new calendar. Kings would even boast of their ability to “count and account”. According to the available tablets of the period, scribes used the sexagesimal place value system, however mathematics was obviously strictly utilitarian. One problem involved deciding on the amount of grain needed to be sown in fields of complicated shapes. Scribes would divide the field into triangular and quadrilateral pieces, and calculate the total by adding these intermediate calculations. Scribes were responsible for computing harvests, and for deciding how much work should be done in a certain period of time. As a worker, if you did not deliver you took over a debt into the next year. If you died in debt, your property was seized, and remaining family members had to pay off the balance.
During the period 1800-1600 BC Babylonian mathematics was divided into tablet-texts and problem texts. Firstly, they had standardised on a simple positional notation system with a base of 60. Oddly there are no addition or subtraction tables, but there are lot of multiplication tables. Again, oddly there are almost no tables of the multiplications of squares of numbers. There are no division tables, instead there are reciprocal tables (i.e. the reciprocal of n is the faction 1/n), and these tables go up to reciprocals of numbers up to several billions. So Babylonians would not divide, they would multiply something with a reciprocal, and there were many tables for this procedure. However, they focused on regular numbers, and gave exercises to find reciprocals only of regular numbers (they appeared to have ignored reciprocals such as 1/7, 1/11, and 1/13). In addition they only used reciprocals where the numerator was 1 (the denominator was to base 60), so an important skill was the ability to find the right reciprocals. If you wanted to divide something by a number, you looked up the reciprocal, and then multiplied your number by that reciprocal. So the skill was in finding the series of reciprocals that when multiplied by your number delivered the correct answer. A simple example might be to find 5/6th of 24 (we know its 20). A scribe might answer that by multiplying 1/2 and 24 and adding that to the multiplication of 1/3 with 24. This is a trivial example, and in fact there were cuneiforms and tables for simple ratios such as 2/3 or 5/6. But you can see the skill that might be required in finding 420 parts of 3600, through finding the right reciprocals. The discovery that Babylonian scribes 'replaced' division by a multiplication of a reciprocal was made ca. 1930. There are a few tables of squared numbers (up to 59), some tables of the square-roots and cube-roots, but there are no tables of the cube of numbers. Reports differ on this, for example, some experts have noted that there is at least one tablet of unknown origin that has cubes up to 32. There were also tables for determining the market rate of goods, and there were conversion tables for weights and measures, and even tables for the ratio of a diagonal to a side of a square. For more information on reciprocals have a look at “Reciprocals and Reciprocal Algorithms in Mesopotamian Mathematics”, by Duncan J. Melville.
The other form of tablets were the problem texts, mostly used in schools. Almost all problems involved computing a number, i.e. no drawing of figures, nor providing proofs. In fact, some experts have suggested that what they did was to use algorithms (sets of rules to be followed), rather than formulas (think algebra and equations). The problems set in the schools involved measuring everyday objects and activities, e.g. weight of..., length of..., area of ..., number of..., etc.
Some of the problem texts were accompanied by a worked example. Other problem text sets a series of questions, all resulting in the same numerical answer (easy to check the right answer). Some of the problems did involve geometric objects, but the answer needed was always a number. Babylonians had no measure for an angle. However some of their problem texts do involve squares, rectangles, triangles, trapezoids, circles, and occasionally cylinders, pyramids, and truncated cones.

For example, let us look at the tablet called Plimpton 322, which consists of 4 columns listing, of all things, Pythagorean triples. These are the positive integers a, b, and c, such that a2 + b2 = c2 (example, 3, 4, 5). These are Pythagorean triangles, and one column is 'width', and another 'diagonal'. It is not clear why they would have listed these triples. Oddly, one column is actually of (c/a)2, which is in trigonometric functions (1/sine2 x), or cosine2 x, the angle opposite to a. Again it is not clear why they listed these values, and why they worked them out for very large numbers, such as 4601, 4800, and 6649. It has been noted that the angle subtended by the sides b and c decreases at a more or less uniform rate as one proceeds down the rows of the tablet (some experts consider this fact purely accidental). We do not know why this was done like this, but the reality is that the Babylonians certainly had some ideas about number theory, and some computational skills to enable them to put their ideas into practice (and all this about 1,500 years before the first Greek mathematicians). In fact Plimpton 322 is often quoted as the oldest document concerning number theory.
Some experts suggest that the Babylonians had a more algebraic than geometric approach to mathematics, and that they may not have viewed this problem as one about the relationship of right triangles. It could be seen as a problem of three squared integers. No matter what, to arrive at the figures it is clear that Babylonians did know how to use second-degree equations (there are clay tablets supporting this fact). This means that they also knew how to use linear equations. Interestingly we use 'x' to represent an unknown, but the Babylonians used sidi, which was also used for the side of a square (and for 'x2' they would also simply use 'square'). In a different text the author said that the word mithartum, meaning “thing that is equal and opposite itself”, was used for both 'square' and 'side of square', and that kippatum, 'thing that curves', was used for both a two-dimensional disc and a one-dimensional circumference.
There is evidence (on clay tablets) that for solving equations they used what is called the 'method of false position', which is an iterative numerical approximation technique. What kind of problems would be solved with these equations? One example would be if we knew the yields (production per unit area) of two different fields, and we knew the difference in absolute production between the two fields, and the area of the two fields together, then we could calculate the area of each of the two fields. Another example was to find the weight of an unknown object. By adding two different known proportions (say 1/7th, then 1/11th), and then weighting them all together, you can calculate the weight of the original object. Of the more than half-million tablets held in different museums and collections, perhaps less than 1,000 involve mathematical problems (other estimates put the total number of tablets at 2 million, including those that have never been analysed). Oddly, mathematical texts are not found everywhere, for example, none have been found in Nineveh (where more than 15,000 tablets have been cataloged). And for the tables of multiplications, etc. the tablets with quadratic equation solutions actually outnumber those containing linear equations. This may simply be due the fact that quadratic equations appeared later in the Mesopotamian time-line. In fact almost all quadratic equations involve generic variables, including length, width, square, surface, height, or volume. A Brief Study of Some Aspects of Babylonian Mathematics is an excellent primer on this general topic.
Just to remind us about how speculative some discussions are about Babylonian mathematics, one author wrote that some books, “included outdated interpretations, gross historical blunders, and fantasies”. What is certain is that the Babylonian place value system was invented about 4000 years ago. And that most the source material for understanding Babylonian mathematics dates from two periods, the First Babylonian Dynasty (ca. 1830-1531 BC), and the Seleucid Empire (312-63 BC), and the vast majority of interesting clay tablets come from the earlier period.
A bureaucratic system run by priests emerged in around 3200-3000 BC in the city of Uruk, and included a system for writing and numeration. The writing served only for accounting. Around 2500 BC city-states saw the appearance of a separate temple-scribe profession, and short royal inscriptions appeared along with very utilitarian mathematics. But in addition there were some examples of supra-utilitarian mathematics, i.e. no relationship to practical needs. A good example is calculating the distribution of barley for workers, for a number far in excess of the working population of the city-state. And on top of that it used the 'divisor' 7, which was never used for genuine practical calculations. By 2350 BC we saw quite a series of supra-utilitarian mathematic problems appear. These involved questions that were not likely to be encountered in the everyday life of a surveyor or tax-collector. It was around this time that metrologies were made sexagesimal, although weight metrology was almost fully sexagesimal from the start.
During reforms around 2075 BC troops of labours were supervised by overseer scribes, who fixed norms for production, work, etc. This is claimed to be the reason for an explosion of table-tablets for every conceivable technical norm, and the use of standard multiplication and division (multiplication by the reciprocal of the divisor) tables. From this time the whole floating-point, place-value system was taught during scribal training. Some experts have noted that the rank-and-file scribes did not learn mathematical problem solving beyond learning the tables by heart. It was at this time that we saw the routine use of 'model documents', emulating real-life documents that a scribe would be expected to produce once on his own. So we know that scribes performed computations, and area calculations, but we know little about how the exact procedures evolved. There were very, very few textual-based test problems, most were of the simple “What is the area of a square of side x?”. Most of the text-based problems from ca. 1900 BC were testing the use of the place-value system. But a new attitude to mathematics did slowly emerge, one with more supra-utilitarian problems demanding some mathematical interest, know-how and know-why. At the same time private letter writing and personal seals became popular with the elite. Scribes were expected to exert scribal abilities beyond just practical requirements. School texts described this requirement and mentioned also mathematics, but gave no particulars. Mathematical problem solving reappeared in between 1800-1700 BC. The dominate form of question was algebraic, solving sides and areas of squares and rectangles. Some supra-utilitarian problems involved the sum of workers, days, and bricks, but a sum that no scribe would ever encounter in reality. We know these types of 'algebraic' problems were being set before Hammurabi became king in ca. 1792 BC. We also see other forms of supra-utilitarian problems involving artificial geometric questions. And in a partial text dated 1775 BC, it is possible that the 'Pythagorean rule' is offered as a general rule (not just for a 3:4:5 triangle). About the same time we also see 'algebraic' techniques applied to supra-utilitarian geometric problems such as partial areas of trapezia and triangles divided by transversal parallel lines. A mathematical compendium found in Tell Harmal lists a large number of 'algebraic' statements about squares (but there were no 'representations' in these Eshnunna texts, i.e. no problems being set). About this time problems started to also appear as riddles, which suggested to experts that these were being set by non-scribal mathematical practitioners, possibly travelling traders. One tablet dating from ca. 1800-1758 BC calculates 30 consecutive doublings of a grain of barley, the first example of the 'chess-board problem'.
At the time there were a number of different cultural centres, each evolving slightly differently, at least according to the available tablets. For example, in Larsa they appeared to have used clay prisms rather than tablets. One particular prism (AO 8862), dated to 1749 BC, tables squares, inverse squares, and inverse cubes.

They also, in opposition to the Eshnunna tradition, did use representations (i.e. practical situations) in their teachings of the 'algebraic' area technique. The Larsa tradition is a good example of the problems facing those analysing clay tablets. Most of the tablets were found by looters and acquired by museums in antique markets, so the entire context of the 'find' has been lost. So the technique is to try to group together tablets having the same age, characteristics, etc., and then suggest a likely place of origin, sometimes this is no better than 'south', other times a city can be suggested.
For example, there are two text groups from Uruk that look mature, and yet are strikingly different. Both groups are very homogeneous, suggesting maturity, yet are strongly different to the point of being unrelated. Experts have suggested two schools of teaching in competition with each other, and an intentional mutual demarcation. One contained long sequences of completed problems (statement and procedure) dealing mostly with right parallelepipedal excavations of small canals (for the important task of irrigation), and they did not announce the results as 'seen' but instead stated the situation in the first person singular (“I have done …") to explain what 'you' should do. The other grouping, dating from ca. 1630 BC, consistently 'see' results, and contained detailed didactical explanations.
In looking at the early evolution of mathematics, experts tend to distinguish between mathematics as an intellectual, supra-utilitarian “end in itself”, and professional numeracy as the routine application of mathematical skills by working scribes.
I mentioned that much rides on the way experts interpret the information on the clay tablets, and we are now going to look at two different ways of interpreting one particular tablet, YBC 6967.

This tablet is written in the Akkadian dialect, and dates from ca. 1500 BC. We start by looking at the analysis of Neugebauer (1945). He writes that “the problem treated belongs to a well known class of quadratic equations characterised by the terms igi and igi-bi (in Akkadian igūm and igibūm respectively). We must assume the product x x y = 60 as the first condition to which the unknowns x and y are subject. The second condition is explicitly given as x−y = 7
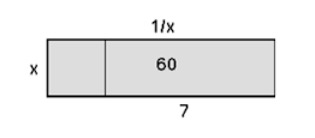
From these two equations it follows that x and y can be found, and in the text a formula is used leading to x = 12 and y = 5”.
Important here is that Neugebauer claimed that equations are “explicitly given” and that the problem is “found from a formula which is followed exactly by the text”. There are not so many people around who can go back to the cuneiform text and are able to check this claim.
For the “explicitly given” equation we read “The igibūm exceeds the igūm by 7”. This indeed corresponds with the equation to the second condition. For the formula we read “As for you – halve 7, by which the igibūm exceeded the igūm, and the result is 3.5. Multiply together 3.5 with 3.5 and the result is 12.25. To 12.25, which resulted you, add 60, the product and the result is 72.25. What is the square root of 72.25, it is 8.5. Lay down 8.5, its equal and then subtract 3.5, the takīlum, from the one, add it to the other. One is 12, the other 5. 12 is the igibūm, 5 the igūm”. Again the text seems to correspond with the formula. There are two minor details here: the ‘lay down’ part sounds a little strange in this context, and Neugebauer added “we have refrained from translating takīlum”, because no sense could be given to it.
In 2002 Jens Høyrup published a book which completely overthrew the standard interpretation of Babylonian mathematics and added a new one. For Høyrup, Babylonian algebra worked with geometric figures. This went completely unnoticed because no figures appear on the tablets. In this problem, the unknowns, igibūm and igūm, are represented by the sides of a rectangle. The term ‘product’ used by Neugebauer should be read as ‘surface’, ‘square root’ as ‘equal side’ or the side of a square surface and adding means appending in length. According to Høyrup the term takīlum which should be read as ‘make-hold’, or making the sides of a rectangle hold each other. Only within a geometrical interpretation, it makes sense to lay down something. The igibūm is now seen as being 7 times longer than the igūm. Cutting that part in half and pasting one of the halves below the rectangle at the length of the igūm we get a new figure with the same surface, equal to 60.

The lower part must be a square, as its sides are both 3 ½. We can thus determine its surface as 12 ¼. The complete figure must also be a square with sides equal to igūm plus 3 ½. We know that the total surface is 72 ¼, the ‘equal side’ of that square therefore is 8 ½. That leads us to a value of the igūm being 5. Placing the cut-out half back to its original place gives a length of the igibūm of 12.
We are presented here with an interpretation completely different from that of Neugebauer. Høyrup accounts for anomalies in the standard interpretation and gives strong arguments for the reading of terms and actions in the geometrical sense. In this new interpretation it makes no sense to speak about equations. Babylonian algebra does not solve equations, as the concept of an equation was absent. But it fits in with our definition of algebra: the method is unquestionably analytical, it uses the unknowns igūm and igibūm and they are represented as abstract entities, namely the sides of a rectangle. No blame is given to Neugebauer for his symbolic reading of Babylonian algebra in 1945. He made a major contribution to the early history of mathematics. The history of mathematics has changed in the past decades and conceptual analyses such as Høyrup’s have become the new methodological standard. In fact today all Babylonian 'algebraic' procedures are now understood as manipulations of lines and areas.
We know that more than half of the problem tablets deal with carrying bricks, building earthen walls, and repairing irrigation canals. All requiring an understanding of plane and three-dimensional geometries, but many of the problems appear to have gone beyond what a practicing scribe would have needed in everyday life. It is odd that finding the square-root was a common problem for students, but there is no record of a practicing 'institutional' scribe ever needing to calculate a square-root.

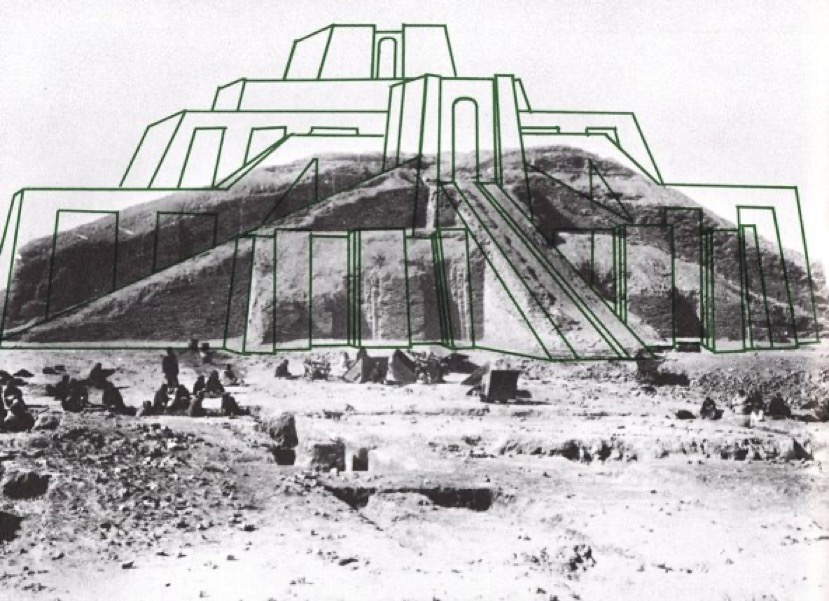
Some experts have rightly noted that a civilisation that was able to plan, build and decorate ziggurats (dating from 2900 BC to about 600 BC), must have had both craftsmanship and an understanding of practical geometry. Towns were planned around these temple complexes, and defensive walls were built (some almost 90 km long). The mud bricks were laid in a herring-bone pattern with mud mortar overlaid with bitumen or lime plaster, which could be smoothed and polished to waterproof them.
Below we have the clay tablet city plan for Nippur, dated ca. 1500 BC. This is through to be the oldest known map ever found.

After the Hittite raid in 1595 BC, the 'Old Babylonian' political system started to collapse. The scribal culture was strongly affected, but literature, myth, ritual and omen 'science' did somehow survive in scribal families. Oddly enough, mathematics did not. Clearly scribes learnt mathematics, but it is also true there appeared to be mathematical tablets and (for want of a better word) non-mathematical tablets. And these non-mathematical tablets only contained very simple numbers presented in the standard sexagesimal system, along with its metrological unit. Was a mathematical scribe in some way different? Did they 'fit in' with the other community of scribes? Who knows?
Moving on to very slippery ground (my understanding is the slippery bit), I have read that the Babylonian mathematical symbols can be divided into those for integer values, those for fractional values, and those signs designating the units of measurement. And of course, a measure is made up of numerical value and a unit of measure (and usually a term indicating the nature of quantified items). It looks simple, but the same (graphical) symbol can have a different meaning depending upon its use, say, one symbol could mean 8 grams or 1/60 litres. This is called polysemy and was common in cuneiform writing. Signs (for quantities) did not have a meaning in themselves, but only in reference to the system to which they belonged. This is very much like how we write today, e.g. “3 kg of honey”. Naturally the Babylonians tried to make things that little bit more confusing by amalgamating the number symbols and metrological unit symbols into one. They would be used in administrative documents just like normal numbers. Examples include a symbol that represented 3,600 m2 and a different symbol representing 21,600 m2, and both followed by the symbol for 'field'. There is evidence to show that measures of capacities remained unchanged, whilst measures of surface area were 'rationalised'. Of course, we do not know the linguistic counterpart for such notations.
We have mentioned the use of numbers in administrative texts. It would appear that in mathematical texts there was a high degree of standardisation with the place value notation (the same symbol used for different orders of magnitude), whereas in administrative texts the notation was more diverse, with different symbols frequently used for the digits 4, 7, 8, 9, 40, and 50. In mathematical texts, numbers had to be with highly standardised positional notation because they were used in algorithms, whereas in administrative texts it just meant what it said, e.g. 7 litres. My understanding is that those writers who had been through scribe school used positional notation, but others who wrote cuneiform texts were less rigorous in their writing of numbers. This might appear of little importance, but in translating cuneiform texts spatial elements in positional notation can change the whole meaning of numerical text. And as far as I can tell, we are not still sure how scribes actually represented spaces on the clay surface. Did they do it differently when writing lines (for algorithms) or two dimensional layouts (for diagrams)? Some experts highlight the fact that we often do not know the difference between 'school tablets' and 'school texts', those copies made by students, and examples of those texts to be copied. In some places school tablets were round, in other places we also have prism tablets made by students. And of course you also had school tablets prepared by teacher-scribes, and we do not know if they fulfilled a pedagogical function or a normative one. Some contained mistakes, but we do not know if they were intentional or not.
Babylonian astronomy (and a lot more mathematics)
Anyway according to most experts, Babylonian mathematics (and possible society in general) stagnated (according to the available evidence) for nearly 1,000 years. Some experts have even expressed surprise that Babylonian mathematics, after its 'swift' creation, did not really evolve for almost 2,000 years, despite periods of great political change.
What can we say about Babylonian astronomy? Were they the worlds first great astronomers? Or were they, as often portrayed by the Greeks, no more than superstitious magicians? The truth is both. During the period after 600 BC Babylonia was no longer an independent state, but had become a province of the Persian Empire. They had adopted the Aramaic language, the lingua franca of the Neo-Assyrians (911-605 BC), the Neo-Babylonians (605-539 BC), the Persian-Achaemenid Empire (539-323 BC), the Parthian Empire (247-224 BC), and the Sasanian Empire (224 BC to 651 AD). In fact, over time many cultures eliminated Sumerian word-signs and clay tablets, and the Elamites, Hurrians, Ugaritans, and Egyptians all developed their own languages and writing systems.
Oddly enough, despite adopting Aramaic and using leather rolls and wooden writing boards, Babylonians had still also retained their clay tablets. In one house dating to about 420 BC, more than 400 clay tablets were found stored in large jars (along with other family documentations, etc.). These tablets were a kind of library with about 30% of the tablets concerned with medical prescriptions and incantations, about 12% on astronomy, astrology and mathematics, and the rest on rituals, hymns, omens, etc. The house owners were some kind of doctor-priest-healer (incantation priests), and the tablets were a mix of fairly comprehensible texts for everything from how to treat diarrhea due to sunstroke to incantations used to ensure normal childbirth. But even with the diarrhea and sunstroke, there is clear evidence that the 'doctor' saw this as the 'hand of god', e.g. god had given the man sunstroke to punish him. From the clay tablets it was also clear that a lot of the fine jewellery found in Babylonian graves were 'charms' with protective properties (semi-precious stones all had different healing powers). Part of this collection of tablets is even more impressive, because about 50 tablets also indicates why they were written, for whom, and who had written them (colophons). And it showed that the role of 'doctor' was passed from father to son over many, many generations. The tablets on mathematics were concerned with reciprocals, lengths, and areas, and there were also a few on time-keeping. Two large tablets listed about 50 mathematical rules and problems about 'seed' and 'reed' measures. The colophon actually says that the tablets were copied from a wooden writing board and that they had been checked against the original. This confirmed that in the period after 1000 BC scholarship was increasingly written on perishable media. Check out “Mathematics, metrology, and professional numeracy” by Eleanor Robson (2007).
We are now in the Neo-Babylonian period (626-539 BC), which was followed by Persian rule with the Achaemenid Empire (550-330 BC). The basic curriculum for a scribe does not appear to have changed much over a period of more than 1,000 years. It is true that texts played a dominant role, and metrological lists became marginalised. At least in terms of the tablet records, but other writing materials were now also in use. Lists of capacities, weights, and lengths were common, but there are no tables with sexagesimal values. In terms of mathematical tables, the only ones surviving are those of the squares of numbers. It looks as if the role of a scribe had in some way become standardised, and that mathematics had moved into the hands of professional scholars (like our incantation priest above) in the big cities of Babylon and Urak (which was later abandoned in ca. 700 AD).
The latest tablets have been dated to ca. 180 BC, and they belonged to an important and prolific scribal family in Urak. This family left a vast amount of documentary evidence, most to do with their work as lamentation priests. They owned a large number of mathematical astronomical tablets, mostly ephemerides, or tables of predictions. The predictions were mostly to do with ominous celestial events, and the correct performance of ritual reactions to them. Their compilation of mathematical problems is the latest datable mathematical cuneiform tablet known. Most of the 17 problems look more or less the same as those found more than 1,500 years earlier, i.e. sums of arithmetic series, capacity of cubic containers, and the sums of reciprocal pairs. The others deal with triangles, squares and diagonals of rectangles, where the presentation is somewhat different, but they are not innovative mathematical subjects. There was much talk of 'seed and read' which were two distinct measures of area, the first 'seed' for agricultural land (including housing), and 'reed' for house plans. At this time 'reed' measurements were dependent upon the number 7, probably as a way to restrict access to urban land measurement by the uninitiated.
By 190 BC there had been a move away from sexagesimal numeration towards the Greco-Egyptian notation of fractions as sums of unit fractions. In a series of prebends, concerning the rights to share in temple income, numbers like 1/6th and 1/9th were added and presented in the Greek-style, e.g. 5/18th. It is possible that those still using tablets and sexagesimal numeration finally became small 'islands' surrounded by a Greek and Aramaic sea.
Before moving on to other topics we are going to follow through a complete Babylonian calculation, showing in as much detail as possible both the procedure and logic used. We must remember that everything is in base 60, and the 0 does not exist.
One common type of problem involved 'excavated pits' in the form of rectangular prisms, and another (below) involved market rates (e.g. commercial mathematics). We will look at tablet MS 3895, which is a so-called 'Old Babylonian' tablet, dated ca. 1900-1600 BC, and from 'the south'. It is a market rate problem where a given quantity of oil is bought and then sold with a given difference between the market rates, and a given profit in silver. The problem is to determine the different market rates and the initial cost in silver. It can be reformulated as a rectangular-linear system of equations, and it is correctly solved. There are only a few mathematical texts dealing with economic matters. The translation of the problem and solution is as follows:
[To bu]y 1 bariga 3 (bán) of finest-quality oil, I borrowed silver from a lending-house, then
I bought, but I did no[t k]now the market rate at which I bought.
I so[ld, b]ut I did not know the market rate at which I sold.
[xxxxx].
[xxxxx].
I accumulated [6 shekels of silver] as profit.
[At what (price)] did I [b]uy, and at what (price) did I sell,
[and] what was the silver?
When [you] work it out:
(You shall) solve (compute) the reciprocal of 6, your profit: you (will) see 10.
You shall let eat each other (multiply) [10] by 1 30: 15
[you will see. You shall note down 45, the difference].
[You shall raise (multiply) 45 by] 15: you will see 11 15.
You shall solve (compute) 1⁄2 of 45 that x x x [you no]ted down,
you will see 22 30. You shall note it down twice,
and (you shall) let them eat each other (multiply), 8 26 15 you will see.
You shall add it on to 11 15: you will see 11 23 26 15.
You shall let go up (calculate) the equalside (square root): you will see 3 22 30.
You shall note down 3 22 30 twice.
22 30, ½ of 45, that xxxxx you noted down,
onto 3 22 30 that you noted down twice
you shall add on to the 1st, you shall tear out (subtract) from the 2nd, 3 45 at which you bought
and 3 at which you sold, you will see.
[You shall solve (compute)] the reciprocal of 3 45 at which you bought, then you will see 16.
You shall let eat each other (multiply) by 1 30: you will see [2]4 shekels of silver. –––––––––––––––––––––––––––––––––––––––––––––––––––––––––––––––––
3 [45 was the rate at which I] bought,
3 [was the rate at which] I sold.
So what we have is a commodity trader who bought a certain amount of the finest quality oil (ì.sag) for a certain amount of silver (kù.babbar = kaspum) at one 'market rate' (mahīrum, expressed in sìla of oil per shekel of silver), then sold it at another market rate. Not known are the invested amount of silver (call it S), the market rate at which the oil was bought (call it m), and the market rate at which it was sold (call it m'). Known are, instead, the capacity measure of the oil (call it C), the difference between the two market rates (m–m'), and the profit (P) earned by the trader through the transaction. The (reconstructed) given data are:
C = 1 bariga 3 bán = 1 30 sìla (where 1 sìla = c.1 liter)
m–m' =⅔ sìla 5 gín/shekel = ;45 sìla/shekel
P = 6 shekels
The unknowns are asked for as follows: m=?, m'=?, S=?
The inverted market rate 1/m, expressed in shekels of silver per sìla of oil, is the 'unit price' at which the oil was originally bought, while 1/m' is the unit price at which it was sold. Therefore, S = C · 1/m is the invested amount of silver, while S' = C · 1/m' is the amount of silver the trader ended up with after the transaction.
Knowing this, it is easy to see that
P = S' – S = C · 1/m' – C · 1/m = C · (1/m' – 1/m).
If this equation is multiplied by the product of the market rates, one gets the new equation
P · m · m' = C · (1/m' – 1/m) · m · m' = C · (m–m').
Consequently, the values of the two unknown market rates can be calculated as the solutions to the following 'rectangular-linear' system of equations:
m · m' = 1/P · C · (m–m') = 1/(6 shekels) · 1 30 sìla · ;45 (sìla/shekel) = 11;15 sq. (sìla/shekel)
m–m' = 45 sìla/shekel
The way in which Old Babylonian mathematicians would solve a rectangular-linear system of equations would be as follows.
If, for given values of A and q,
m·m' = A and m–m' = q, then
sq.p/2 = sq.q/2 + A, where p = m+m'
This is a modern-type equation in symbolic notation, but the Old Babylonian counter-part was a piece of 'metric algebra', where m and m' were interpreted as the sides of a rectangle and where the product m · m' was interpreted as the area of that rectangle. If four such rectangles are put together to form a ring of rectangles, then p = m+m' is the side of a square bounding the ring from the outside, while q = m–m' is the side of a square bounding the ring from the inside. Therefore, the Babylonian geometric counter-part of the modern equation above is the straightforward observation that the area of the square with the side p/2 = (m+m')/2 is equal to the area of a square with the side q/2 = (m–m')/2 plus the area of the rectangle with the sides m and m'.
In the metric algebra solution procedure in MS 3895, which started with the computation of the product m · m', the rectangular-linear system of equations for the unknowns m and m' is solved in the following series of computations (here the terms are expressed in quasi-modern symbolic notations and sexagesimal numbers with semi-colons):
(m–m')/2 = ;45/2 = ;22 30, two copies of ;22 30 are noted down sq. (m–m')/2 = sq. ;22 30 = ;08 26 15 sq. (m–m')/2 + m · m' = 11;15 +;08 26 15 = 11;23 26 15 (m+m')/2 = sqs. 11;23 26 15 = 3;22 30, two copies of 3;22 30 are noted down
recall that (m–m')/2 = ;45/2 = ;22 30 was noted down twice
m=(m+m')/2+(m–m')/2=3;22 30 +;22 30 = 3;45 (sìla/shekel), and
m' = (m+m')/2 - (m–m')/2 = 3;22 30 - ;22 30 = 3 (sìla/shekel)
1/m = 1/3;45 = ;16, S = 1/m·C = ;16 · 1 30 = 24 (shekels)
The main result of the computations, giving the values of the two market rates, is repeated below the horizontally drawn line seen above.
I am certain that the above example was not easy to follow in all their details, but we can see how Babylonian scribes were taught to address the problem, and what mathematical techniques were at their disposal. I must stress that the experts who worked on this type of text first had to understand what was written, then understand the notations, understand the figures to base 60, and finally understand the underlying mathematical logic. Just as an example, the ablative suffix '-ta' (not commonly found) actually indicate rate per unit, and that unit is implied to be 1 silver 'gin'. Also the verb 'sa' probably means making the equivalence of one item, expressed as a quantity in a standard metrological system, to another item, expressed as a quantity in another standard metrological system. In our case we are talking about the buying of oil with silver and the sale of the oil for more silver. The verb 'bur', as opposed to 'sa', denoted the (re)sale, but in both mathematical and administrative text it is not used. In mathematical texts the verb 'pašārum' means both 'to solve' and 'to sell'. Turning to the units of measurement, some units are difficult to translate because it depends upon the age of the tablet, and thus the degree of standardisation of notations of units of measurement at that time. For example, shekel is translated as a unit of weight, however in older texts, 'gin' could have been a weight or a unit of capacity, or even of surface. I could go on, but I think the point is made. Before even looking at the mathematical procedures used by the scribes, experts needed to understand the questions asked. If you have a passion for this topic have a look at “Interest, Price, and Profit: An Overview of the Mathematical Economics in YBC 4698”.
Before moving to Babylonian astronomy, we are going to look rapidly at the algorithmic nature of Babylonian mathematics. We know that they initially adopted a floating-point sexagesimal numbering system, but not including any exponent part. Thus a number such as 2,20 could stand for either 2 x 60 + 20 = 140, or 2 + 20/60 = 2 1/3, or for 2/60 + 20/3600. Even a simple 1 could mean 60 or 15, or even ¼. Scribes had to keep track of not only magnitudes but also the associated metrology, since numbers were often not associated with a unit of measure or a quantifying term. Babylonians possessed addition, subtraction, multiplication, and division, and they could process many types of algebraic equations through algorithms, or step-by-step lists of rules, a kind of hidden language representing the formula. Instances of algorithms without numbers are very rare, but one such tablet reads:
Length and width is to be equal to the area
You should proceed as follows
Make two copies of one parameter
Subtract 1
Form the reciprocal
Multiply by the parameter you copied
This gives the width
In other words, if x + y = x x y, it is possible to compute y by the procedure y = (x - 1) - 1x. The fact that no numbers are given made this passage particularly hard to decipher, and it was not properly understood for many years, hence we can see the advantages of numerical examples.
When we talk of algorithmic procedures we have to recognise that those used by the Babylonians were almost all related to 'simple' calculations. They did not have conditional branches ('go to, if'), nor a 0, nor negative numbers. They did not use iterations, but they were familiar with linear interpolation. During the Seleucid Empire (312-63 BC) most of the mathematical tablets deal with astronomy, rather than what might be called pure mathematics. However, a symbol for 0 was used instead of an empty space, and there were formula for the sum of geometric series and the sum of quadratic series. An author called Inakibit-Anu (ca. 200 BC) built a remarkable table (tablet AO 6456). There are exactly 231 sexagesimal numbers of six sexagesimal places or less which have a finite reciprocal and which begin with 1 or 2 (they were called regular numbers). His table contained all of them, and thus his table is considered the earliest known example of a large file that has been sorted. So Babylonian tables were, in many ways, general procedures for solving whole classes of problems. In fact, many tablets conclude with “This is the procedure”. We should also not forget that the operations made with positional numbers actually refer to algorithms. Those linked to reciprocals, square or cube roots usually have traces left with the texts, whereas others (i.e. multiplication) have left few traces in the texts found on tablets. In these cases scribes would perform their calculations from memory, and with the help of multiplication tables. As such Babylonian procedures are genuine algorithms, and their habit (a very useful habit) was to explain their algorithms by example.
Babylonian astronomy (finally)
Most authors see Babylonian mathematics developing hand-in-hand with Babylonian astronomy, dating from about 1800 BC. The most important work occurred from around the 7th C BC, when they linked together mathematics, astronomy, and medicine through a new device we call the zodiac.

The tablet on the left dates from around 800 BC, and records astronomical phenomenon such as eclipses, the position of the planets, and the rising and setting the Moon. The other tablet records the passage of Halley’s Comet in 164 BC. In fact the city of Babylon recorded astronomical phenomenon on a month-by-month basis for about 700 years, starting from the 8th C BC (they probably started to record eclipses, etc. as early as the 13th C BC). It was on this basis that they were able to develop increasingly accurate models for predicting the movements of heavenly bodies. The details on the rising and setting of the Sun, Moon, and five visible planets is truly impressive, and are considered to be the oldest scientific documents in existence. And in addition those records taken over 700 years, make it the longest continuous scientific record in all history. It was sometime in the 5th C BC that Babylonians divided the night sky into twelve equal sectors, the zodiac. They also gave us many of our modern-day zodiac references, e.g. the Moon could be in the 12° Bull, or the Sun was in the 29° Crab.
Why go to all the trouble of recording astronomical phenomenon on a month-by-month basis for 700 years? One answer is that medical practices were often based upon knowing the configuration of the stars and planets at the time someone fell ill. This would enable a 'doctor' to suggest the right healing materials, e.g. amuletic stones, aromatic incenses, and healing plants were all associated with different zodiacal constellations.
Another related answer is that if the gods had created the world based upon some fundamental principles, embedded with complex mathematical patterns describing the movement of heavenly bodies, then maybe Man could understand them. Would that not give Man a richer, more full appreciation of the gods, and a better idea about Man’s place within creation. If the gods controlled the sky according to some set of sophisticated rule, perhaps they also controlled Man with the same rules (thus all the creation myths and elaborate cosmogony theories).

This is an Assyrian star planisphere (K 8538) probably dating from the period 668-627 BC. The actual purpose of the 13 cm diameter clay tablet is uncertain, but it may have had some 'astro-magical' purpose.
It is true that both the Egyptians and Babylonians were practical cultures. Their cosmologies were practical, just as was the mathematics and geometry the practiced. But their creation myths were also at the core of their religions (see Babylonian religion and Ancient Egyptian religion). From them we have our 12 zodiac signs, our lunar calendar, and the solar calendar with 12 30-day months, and a 365-day year. Principal deities were heavenly bodies, so it was important to know where and when they appeared and disappeared, i.e. the pre-eminent Egyptian god was the Sun god, Ra. But it is said that neither cultures created a cosmological model to describe their observations and practices, and it would be up to the ancient Greeks to propose around 600 BC the first cosmological models (see this timeline).
For the Babylonians, this may be because our record of their world view is incomplete, but it appears that they did not link astronomical observations with thinking (albeit limited) on cosmology. Yet they did see the Earth and heavens as having a round shape, and they also thought that the Earth was not in the centre of the universe. However they did place the Earth in a centre of a sphere to which the stars were fixed. The motion of Sun, Moon and planets was then studied in relation to the background of the stars as a fixed frame of reference. In fact Babylonians had a plurality of heavens and Earths, there was the heaven of the stars, and above that 2 more heavens, and they had an Earth (Ma), an underground of the Apzu (primeval sea), and an underground for the dead (Kur). I have also read that they had seven heavens and seven Earths, probably linked to the idea of creation by seven generations of gods.
Examples of astronomical observations exist with tablets divided into two halves, one part for astronomical observations, and the other for acts and facts. In terms of astronomical observations, they recorded the positions (rising and setting) of the Moon, eclipses, solstices, equinoxes, and the position of the planets. Acts and facts would be anything that the priest-scribes thought might be affected by celestial omens, e.g. the level of the river, the prices of commodities, and political events. For example, when Alexander the Great defeated Achaemenid Persian Darius III (in the Battle of Issus, 331 BC), there was “a lunar eclipse, the west wind blew, and deaths and plague occurred”. The next day the sky was covered in clouds. And on the 3rd day a meteor flashed past, in an otherwise cloudy sky. The lunar eclipse was also confirmed by both Greek and Latin sources. But the Babylonians went on to note the price of barley, mustard, and sesame. They also noted the position of all the planets, and the level of the river. Was the victory of Alexander a surprise? No, Babylonian priest-scribes had already predicted for the lunar eclipse that “the King will be purified for the throne, but will not take it”. An intruder from the west will come and exercise his kingship for eight years. He will conquer his enemies, and bring abundance and riches. He will pursue his enemies, and his luck will not run out. Later we would learn that an eclipse on the 13th brought evil for Babylonia, and the west wind implied that doom was to come from the west. Saturn being visible meant that the omen was urgent, and Jupiter, who could have neutralised it, had set. In fact Darius III did what all self-respecting kings did, he appointed a substitute king for a few days. This 'King' would bear the brunt of the gods’ wrath, and Darius III remained unharmed. Once the battle was finished, the 'King' was swiftly killed. But Alexander’s luck did run out 8 years later, when he died in 323 (despite appointing a substitute King).
Whilst we have learned a lot from the Babylonian tablets themselves, after ca. 600 BC we also learn a lot about Babylonian practices from later Greek texts. In fact Alexander had the Chaldean cuneiform texts translated and sent back to his scientific advisor Callisthenes (ca. 360-328 BC). The tablets of the Chaldeans are often translated as diaries, but the real sense was double, meaning both “observing” and “guarding”. The astronomical diaries, and the Babylonian Chronicles that exploited the data collected, described impartially only what had happened, not how or why. It was Herodotus (ca. 484-425 BC) who would add causality and a historiographic narrative to the collection of facts, and who would be given the title “The Father of History”. But in fact the 'additional' data collected by the Chaldeans does shine a light on to that period. For example, the low price of commodities showed that Darius III certainly knew about logistics and looking after his people, whereas with Alexander prices for commodities shot through the roof. Some experts have suggested that the Chaldean records show that prices could have been affected by a “little ice age” that occurred during the reign of Alexander. The records also show that the armies of Darius III were deserting on the eve of the battle, so Alexander’s victory was over a fleeing army. This is quite different from the 'classical' Greek story of a great victory. Even today new facts are being discovered about Babylonian history, showing that the Chaldean diaries remain an invaluable historical source.
On a more practical level, Chaldean (ca. 10th C BC) astronomers would discover the 18-year (6585 days) Saros cycle, used to predict the lunar and solar eclipses. There are strong suggestions that after about 300 BC Neo-Babylonian astronomers were developing predictive models so that they did not need to consult past records. However most experts think that they continued to focus on using ephemeris, diary journals of astronomical observations, rather than adopt a theory. Recorded Babylonian predictions are all based upon empirical data and arithmetic, although there is some evidence to suggest that they used some geometric methods from ca. 350 BC. Those Chaldean astronomers also collected together omens originally from the 2nd millennium BC. These omens referred to things that were seen to have been linked to the appearances of the Sun, Moon, and planets, as well as meteorological phenomena. A typical omen might be, “if Jupiter rises in the path of the stars, the king will overthrow his enemies in battle”. We also know that by the 3rd C BC Babylonian had procedural texts listing the rules for computing ephemerides (daily positions of the Sun, Moon, and planets) used for navigation. What we see with the Babylonians is a odd mix of sophisticated calculations based upon limited observation skills. For example they knew that Jupiter moved across the sky slower than the Sun, they knew that Jupiter would rise roughly every 399 days (the 'mean synodic period'), and they knew that it would successively rise in different regions of the sky corresponding the zodiac signs (the 'mean synodic arc'). They even registered that both the Sun and Jupiter would return to essentially the same positions after 427 years, and then the cycle would repeat itself.
Unlike the Greeks the Babylonians did not use a geometric model with the sky as a sphere and the Earth in the centre, they used numerical schemes and rules for computing the position of the Sun and Jupiter. Yet, at the same time, their records were based upon crude observational skills. The scribes had neither instruments to observe the precise position of the Sun, Moon or planets, nor did they have a coordinate system for recording them. The records just say Jupiter was 'in Leo'. It has been shown that it was possible to go from crude observations to useful ephemerides using a set of standard rules, but there is no record of these rules, or their use. Babylonian astronomy, along with arithmetic and geometry, was the first of the natural sciences. The way the Babylonian scribes applied mathematics to describe and understand complex natural phenomena in many ways laid the foundations for our modern world. On a much more prosaic level, we must ask the question, why did they bother? According to many experts, the answer is simply bad weather. The records also showed when rain and clouds made it impossible to observer the night sky. Therefore from bad weather was born their desire to find the periodicity of the night sky, through observation, recording, and developing predictive techniques. There is much speculation in all this since there are no records of any theoretical analyses by the Babylonians, just descriptive texts and almanacs. This has not stopped some experts claiming that it was the Babylonians who were at the origin of rigorous, technical science, and not (the intellectually less challenging 'fables' of) the Pre-Socratic philosophers!
In discussing Greek and Babylonian astronomy, their objectives were the same, i.e. use empirical data and be able to compute the positions of celestial bodies for any given moment. The Greeks invented an intermediate step, by constructing a dynamic model based on circular movements. It is from this model (and the empirical data) that they created their navigational almanacs. It would appear that the Babylonians did not do this, preferring to try to obtain their tables purely on mathematics grounds and based upon their empirical data. So they had the data and just looked for simple periodic functions that fitted their observations. Their simplest tables were just observations for each synodic month, the period of the Moon’s phases, and a linear interpolation giving a kind of zigzag. There is evidence that the Babylonians used different ways to represent periodic functions, all with the aim of avoiding the sharp change of direction at the extrema. Rather than try to find a complex model of celestial movement that came close to reality, the Babylonians just looked at different features of the same celestial body as if it were several independent celestial bodies. For example, Mercury was seen as the morning star and as an independent evening star, and in fact it was treated as being 6 different “planets” together presenting a very satisfactory representation of actual movement and day-to-day positions. As such the functions that were said to describe the movements of a planet were intellectual abstractions that had little to do with observed phenomena. They predicted when an eclipse or partial eclipse might occur, but for the rest of the time the predictive functions were useless. We have to remember that astronomical events were the important thing, since they were presumed to exert an influence over peoples everyday lives. And these arithmetic functions did the job. Recently (Jan. 2016) it has been suggested that there was more to the use of these intellectual abstractions than first thought. In an article in Science it is suggested that Babylonian astronomers modelled the 60 day passage of Jupiter using two trapezoids. The author says that the tablet inscriptions reflect “a more abstract and profound conception of a geometrical object in which one dimension represents time”.
Time measurements of the Babylonians depended upon the season (i.e. 'seasonal hours'), since they divided the day and night into 24 equal hours, starting at sunrise. So an hour depended upon the length of daylight, and their lunar theory depended upon predicting the evening of the first visibility of the new Moon (it defined the new month and thus the whole year). The Greeks developed spherical astronomy (i.e. spherical trigonometry) to deal with predicting the new Moon, the Babylonians tried arithmetical schemes to predict the rising times of the zodiacal signs as a function of longitude.
Another important role for Babylonian astronomy was to regulate the calendar, and thus the proper time for crop planting and land irrigation. Astronomers (probably around 747 BC) recognised that 235 lunar months were almost identical to 19 solar years, and they concluded that 7 of the 19 years should be leap years with an extra month. A standard system was introduced in 503 BC, in such a way that their new year was always close to the first day of spring (vernal equinox in late March or early April). This involved adding an additional 6th or 12th month. This fact is first seen in royal commands sent to the temples, other intercalated months were announced by the temple priests. Intercalations became standardised in ca. 367 BC. A total of 80 intercalated months have been found. In 331 BC the calendar was changed to a 76 year cycle, and when Alexander captured Babylon he imposed this new calendar on the Greeks as well. This new calendar has been attributed to Kidinnu, a 4th C BC Chaldean astronomer, who is also said to have discovered that the 251 synodic months are identical to 269 anomalistic months. Kidinnu is also said to have discovered the Earth’s precession, the slow reorientation of the Earth’s axis (but many experts now attributed that discovery to Hipparchus).
If you are interested in the topic of Mesopotamian Astronomy and Astrology, there is no better place to start than this bibliography (but I do not know if it is maintained and up-to-date).
We must presume that early on astronomy was probably a technical branch of religion, and predicting the movement of the plants using mathematics was in the hands of priest-scribes. The movement of the five visible planets, and the Sun and Moon, and the stars all represented the activity of their corresponding gods. So 'reading' the heavenly motion of celestial bodies would enable priests to predict what was about to happen on Earth. And 'reading' was really about weighing up precedents, based upon detailed records of the what had happened in the past. Questions concerning the nature of the planets, the source of energy of the Sun, or the way celestial bodies might be linked together were irrelevant. Religion would have taken the results of astronomy, and presented them as astrology (and Babylonian astrology was already well developed by ca. 3000 BC). Some experts have thus concluded that Babylonian astronomers did not try to find objective truths to explain their observations, thus they were not being scientific, and therefore their astronomy was not scientific either.
Based upon the idea that astronomy empowers astrology, which then influenced the actions and history of Man, it was natural to assume that it would be easier to study astronomy by observing Man rather than distant stars.
On the other hand Babylonian astronomers made careful observations of the night sky, and they kept excellent records over many, many generations. They could not fail to notice that many astronomical events recur periodically, enabling them (from about the 7th C BC) to predict how the heavens would look at some point in the future. Again experts think that this desire to predict was less motivated by a desire to understand, and more likely to be response to royal demands for better forecasting. However making predictions is a key element of any scientific method, as are careful measurements and record taking. On the other hand, it is true that Babylonians still 'explained' an eclipse using gods and demons, and not the physical movement of celestial bodies across the Earth’s sky. It is not at all clear if Babylonians thought too much about why the Sun emanated heat and light.
We know that the Earth spins like a 'top', rotating on its axis once each day, and at the same time slowly precessing, thus presenting a slowly changing night sky. It would appear that Man was aware of these slow changes, but we are not sure how. The estimate of a period of ca. 26,000 years was made by the Greek Hipparchus of Nicaea (ca. 190-120 BC), possibly using the extensive records of Babylonian astronomical observations. This precession of the Earth’s axis gives rise to a slow change in the position in the which the Sun appears to rise each year. The Sun rose in Taurus until ca. 1900 BC, then Aries until ca. 1 AD, before entering Pisces (Christ the Fish). The Age of Pisces will terminate ca. 2150, and be replaced by The Age of Aquarius.
So by 300 BC astronomers were able to make accurate predictions of solar and lunar positions, and the sun dial was the instrument of their understanding.
Babylonian lists and omens
So far we have written about Babylonian mathematics and astronomy. In each case we have mentioned the lists they created, and the calculations they performed. However making a list in itself was a scientific endeavour for the Babylonians. Sumerians invented their writing system cuneiforms, initially as pictograms, and perhaps by 3000 BC as a written form of the Sumerian language. Their writing system was adapted for the writing of other languages, including Akkadian (sometime after 2900 BC). In fact it is said that an intimate cultural symbiosis developed between the Sumerians and Akkadians, including widespread bilingualism (some say even a linguistic convergence). Akkadian gradually replaced Sumerian as a spoken language ca. 2000 BC, but Sumerian did continue as a sacred, literary and scientific language unit ca. 100 AD. After about 800 BC Aramaic started to replace Akkadian.
As the writing system evolved into signs having a link with spoken words, so lists could be arranged as word lists. From ca. 3000 BC distinct lists appeared, primarily containing compound words, for objects made of wood, metals, reeds, leather, stone, wool, etc. Other lists were made for plants, including trees and grains, and for wild and domesticated animals. And there were list of body parts, geographic names, stars, and gods. These were practical lists, and practicalities remained a key feature in the scribe schools. Order was seen as the work of the gods, and ordered lists, etc. were a way to see the gods at work. Certainly through to ca. 2400 BC the Sumerians did not connect things together through ideas about nature, theology, mathematics, law, or other 'abstract' thoughts. They had no conceptual framework for what we would call 'natural laws', and their lists were essentially one-dimensional. The best they could come up with was order based upon their mythical literature, or tablets listing their kings. When several kings ruled over different parts of the region at the same time, they were listed one after another, and not side-by-side. And historical and mythical kings were mixed up in the same lists. Even with the emergence of mathematics, one-dimensional lists were just replaced by one-dimensional tables.
When the Sumerian language started to mix with the Akkadian language and the Eblaite language, sometime between 3000-2500 BC, two-dimensional lists were created of two different languages. These bilingual lists became the first lexical aids in human history. Around 2400-2200 BC we have Sumerian-Akkadian lists of grammatical forms, even if they were learned by examples rather than through rules. Synonyms and homonyms were collected, which only highlighted even more that between Sumerian and Akkadian many words were not exact equivalents. By 1400-1200 BC four-column lists had appeared to cover 4 different languages, e.g. including Hittite, Ugaritic, and Hurrian, but, oddly enough, not Aramaic.
So until the appearance of multi-column lists, the one-dimensional list was a kind of inventory, listing objects or living creatures. This did not stop them developing the lists, for example, adding obligations and explanatory notes. Trees were treated extensively, and other plants were on other lists. Domestic and wild animals came before birds and fish. Some tables had so complex explanatory additions, that you needed some preliminary instructions before reading the list. With the advent of 2-column lists, and by 1200 BC, lists of Sumerian and Akkadian gods became, at best, confused. The relationship between the different gods became unclear, and variations in names appeared for what was in effect the same god. Some of the originally independent gods 'evaporated', or were 'reinterpreted'. Some gods just got absorbed into other gods. There were also some 2-column lists of astronomical instructions. We must remember that astronomical observations were linked to 2-column lists of pharmaceutical plants and stones. And these were linked to 2-column lists of flora, fauna, and mineral drugs, in most cases without any instructions or comments.
Babylonian lists were typical of Babylonian thinking. They were lists or compilations of facts. They represented knowledge in detail, but lacked a formulation in terms of basic principles. Yet it is through these lists that we can see the Babylonian sciences. Their most important science was the one of omens. Omens were compiled and listed, along with their interpretations, and we are talking of them listing and ordering 10,000’s of omens. Each omen started with a “When ....” and concluded with a “What” (trouble, sickness, etc.). These lists were ordered in a very strict way, since omens could occur simultaneously, or be related one to another. Initially omens were encounters with animals, or eventually were linked to anomalies of birth, but rarely linked with the planets and stars. Sheep livers would be inspected, the way oil flowed over water in a basin, or the way smoke rose and curved. All would be omens. Most of the questions were about military campaigns, or public offices, and the answers were usually partial 'yes/no'. The 'yes' and 'no' were counted, and the bigger number was the answer. Individuals were interested in family relationships, house and property, and recovery from illness. The sheep liver divination lists passed down in to the Hellenistic period (the Bārûtu was a compendium of sacrificial omens). Terrestrial and astrological omens dominated through to ca. 1000 BC. There were 10,000’s of these, plus 1,000’s of birth omens, calendar omens, and diagnostic omens. And we should not forget the 1,000’s of physiological and dream omens.
Omen collections represent possible the most precise set of observations made by the Babylonians. A sheep’s liver would be observed, registered and recorded on clay tablets, as would births and miscarriages. The body structure of animals would be observed in detail, and in particular for snakes. Through this we see the zoological knowledge of the Babylonians. Weather omens were also important, and incorporated in to astrological omen series. Man was studied in detail. Moral values, as well as outbursts of temper, were all interpreted in terms of good or evil omens. Dreams were recorded in great numbers, and from dreams, sicknesses were diagnosed.

These tablets are two of a collection of 70 dealing with Babylonian astrology, and the bulk is a collection of omens as interpretations of a wide variety of celestial and atmospheric phenomena relevant to the King and State (used as astro-meteorological forecasts). The tables date from ca. 650 BC, but some of the omens date back to 1646 BC.
Of course the gods always played a role in Babylonian society, but it was only with the 2-column lists that we learned a little about the relationship between different gods, and a little about their associated rituals. After ca. 1000 BC, cultic commentaries were added to the lists, however they were more like colourful pronouncements than useful information. Prayers and mythic poetry are more reliable sources of information, but lack a systematic viewpoint. Politics can be occasionally seen, as one or other dynasty disappeared or reappeared on different lists. After about 1100 BC some lists included wars covering also the previous 250 years or so. The Babylonian Chronicles recorded major events from 747 BC, and from about the same period astronomical diaries also contained short historical reports.
It is only during the Seleucid period (312-63 BC) that the fate of individuals was determined by the planets and stars. After 1200 BC astrological observations started to be collected, and as a significant number of observation emerged so did their use as omens. Initially the fate of the state, cities, or noble families were determined by such omens. For example Enûma Anu Enlil was a collection of celestial omens dating from sometime between 1595-1157 BC, but some of the omens certainly date from earlier periods. The collection covers 70 tablets, and the author thought that the gods had created the movement of the plants to give people indications about the future. So priests observed the skies, collected data, discovered irregularities, and warned the authorities when something bad was going to happen.
So these lists give us a sense of Babylonian understanding in the 'natural sciences'. From this we know about how Babylonians saw land animals, insects, and worms. We know less about birds, and even less about fish. The body parts of animals are treated along with those of people. Part of the problem was that initially one word might represent different animals, e.g. dogs, wolves, and predatory cats all had initially the same name. Flora, fauna and minerals having therapeutic value were extensively listed. Many medicinal herbs had names from foreign lands. From about 1000 BC, medicinal plants and stones were describe well enough to be recognisable. In many cased the parts of the medicinal plant are described, including the roots. Flora and fauna occurred the most in terrestrial omens, e.g. behaviour of animals, location of plants, etc. Some texts actually even described birdcalls in a vocalised form. Classification of body parts or animal behaviour is missing, but what is there shows an intense sense of observation. The problem is that lists of omens tended to mix up actual observations with invented ideas, some evidently absurd, others less so.
Experts tell us that Babylonian physics and chemistry was almost totally non-existent. At least in terms of tablets and lists. Of course they knew about the lever, elements of metallurgy, and the making of different kinds of glazes and cosmetics. There were procedures and formulas for making these things, but not written in a way that we would consider scientific.
Babylonians studied groups, communities and individuals. The interest in groups dates from the earliest times, and laws and collections of laws governing groups of people were some of the earliest textual examples. There were tablets of how best to conduct affairs of state, but we cannot find anything on the fundamental nature of laws, nor on what is today called political science. 'Wisdom texts' existed, and touched on some philosophical, pedagogical and ethical questions.
Omen collections did deal with the individual, and there was an interest in the anatomy and physiology of the human body, in so far as it dealt with healing illnesses. As compared to the Egyptians, surgery was not a major technique in Babylonia. The doctor worked primarily with medication taken orally, and along with some magical incantations and prayers. We still have not understood and translated many plants, minerals, and diseases. Diagnostic texts, prescriptions, and descriptions of symptoms are very limited, but from about 2300 BC things improve substantially. But we have to wait until ca. 700 BC to see appear the great medical compilations, and reports on individual cases. From this period we have diagnostic omens with a few symptoms, with statements on whether the disease can be healed, or how long will it take before death. These are often called “the hand of the god” texts, with some gods being more malevolent than others. The other texts are for individuals, and are extracts from larger compilations. What is important is that quite a few of Babylonian medicaments are similar to the ones we use today. However we have not found a physiological theory developed by the Babylonians to describe the functioning and effects of their medicaments.
I mentioned above 'wisdom texts', but most experts prefer the term 'works of guidance', in that they were moral exhortations of so-called 'Counsels of Wisdom'. These ranged from “avoid bad companions” to the “undesirability of marrying a slave girl”. But they also included positive moral statements such as “maintain justice to your enemy”, “do not insult the downtrodden”, and “speak well of people”. After ca. 1500 BC dialogues appeared that told a moral story. These types of 'wisdom texts' were also common in Egypt about the same time, suggesting that everyone needed wisdom literature, and in fact through history this type of literature is one of the most common. In many ways these “wisdom texts” build a foundation for the emergence of philosophy.
Colour on clay tablets
A last point on this webpage more or less dedicated to clay tablets and cuneiforms concerns colour. We know that the tablets themselves could be a bone white, chocolate or charcoal colour, and even occasionally red, green or purple depending upon the clay matrix, the site of the original clay deposit, and the firing process.
What is even more interesting is that today we can bolden, underline, highlight, and even animate our texts, in order to emphasis a message. But what could the Mesopotamians do to emphasise their cuneiform texts?
Raman spectroscopy is a wonderful non-destructive technique to identify the fingerprint of trace molecules found in paints, ceramics, etc., and it has been used to look at some cuneiform tablets dating from ca. 2600 BC. This type of tablet would have been made in wet-clay and after 'carving' dried in the sun (i.e. not exposed to high temperatures). Pigments were detected in two tablets, one on the deeds of the gods and the other on the account balance of certain products. What the specialists found were traces of yellow (Crocoite, Lead-Tin), white (Quartz, Titanium Oxide, Lead, Calcium Phosphate, Gypsum), and red (Hematite, Cuprite) pigments. And even an orange from a mix of Lead-Tin Yellow, Gypsum and Cuprite red. Most of these pigments were already known for pottery decoration, however the presence of lead-based compounds was new (even if lead compounds were known in ancient Mesopotamia). So what is evident is that the Sumerians did colour their clay tablets, mostly in the engraved signs, but also over the tablet surface. It appears that the pigments were applied when the tablets were still soft, possibly during the carving, i.e. pigment ingredients are mixed with the quartz micro crystals in the damp clay surface.
We don't know why the tablets were coloured with pigments, however we do know that red dots were placed on tablets to 'highlight' complete rows in lists. In other cases red dots were also used to separate units from poems. In this particular tablet red was used to 'highlight' the sum of credits and debts. On the other tablet yellow appears to have been used to 'highlight' a deity.